|
Dado
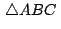 un triangulo cualquiera. Se
construyen exteriormente los tri�ngulos equil�teros un triangulo cualquiera. Se
construyen exteriormente los tri�ngulos equil�teros
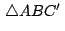 , ,
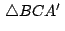 y y
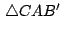 . Bajo estas
condiciones se cumple que: . Bajo estas
condiciones se cumple que:
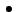 Los segmentos Los segmentos
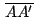 , ,
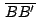 , ,
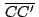 son congruentes. son congruentes.
Se deduce que los tri�ngulos
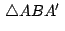 y y
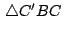 son congruentes por tener son congruentes por tener
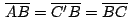 y y
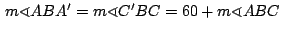 , de donde , de donde
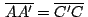 . .
De forma an�loga son congruentes
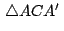 y y
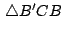 por lo que por lo que
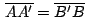 . .

 Los c�rculos circunscritos a los tri�ngulos Los c�rculos circunscritos a los tri�ngulos
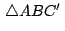 , ,
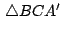 , ,
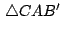 pasan por un
mismo punto. pasan por un
mismo punto.

Si
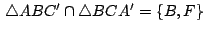 ,
como ,
como
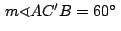 se tiene se tiene
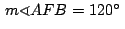 y como y como
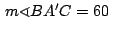 se tiene: se tiene:
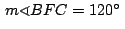 por lo
que por lo
que
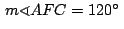 y como y como
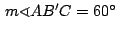 se deduce que el cuadril�tero se deduce que el cuadril�tero
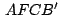 es inscriptible de donde es inscriptible de donde
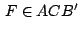 . .
Para completar la demostraci�n debemos considerar el caso en que
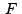 es exterior a es exterior a
 y tambi�n el caso en
que y tambi�n el caso en
que 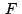 coincide con coincide con 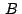 . .
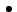 Las rectas Las rectas
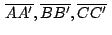 son
congruentes en son
congruentes en 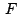 y forman y forman 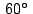 entre ellas. entre ellas.

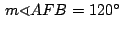 y y
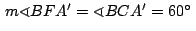 por ser �ngulos inscritos que
abarcan la misma cuerda por ser �ngulos inscritos que
abarcan la misma cuerda
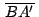 de donde: de donde:
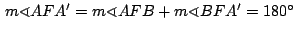 por lo que por lo que 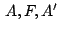 est�n
alineados. est�n
alineados.
De forma an�loga 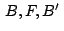 y y 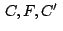 est�n alineados. est�n alineados.
La demostraci�n quedar� completa considerando el caso en que
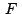 es exterior a es exterior a
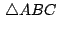 y tambi�n el caso en
que y tambi�n el caso en
que 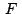 coincide con coincide con 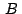 . .
Revista digital Matem�tica, Educaci�n e Internet.
Derechos Reservados
|


