Cuando los elementos an se acercan a
cierto número real l, conforme n se hace grande, decimos que la
sucesión
 an
an converge a l. Para precisar esto
mejor, tomamos un intervalo
]l -
converge a l. Para precisar esto
mejor, tomamos un intervalo
]l -  , l +
, l +  [, con
[, con
 > 0. Si los an se acercan a l, debe tenerse
an
> 0. Si los an se acercan a l, debe tenerse
an 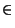 ]l -
]l -  , l +
, l +  [, para n suficientemente grande. Esto
justifica la siguiente definición.
[, para n suficientemente grande. Esto
justifica la siguiente definición.
Definición 2.6.2
Decimos que la sucesión (
an) converge a
l 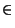 IR
IR si:
Para todo
 > 0 existe
N
> 0 existe
N 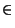 IN tal que
IN tal que
En tal caso decimos que la sucesión (
an) es convergente, y
que su límite es
l. Escribimos
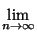 an
an =
l.
También se usa la notación
an 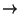 l
l.
Si no existe tal
l, decimos que (
an) diverge.
Ejemplo 2.6.12
La sucesión constante
(
c) =
 c
c,
c,
c,...

converge a
c.
En efecto, como
 an
an -
c
= 0 <

para cada
n, la
definición de convergencia se cumple trivialmente.
Ejemplo 2.6.14
La sucesión
 an
an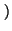
definida por
an =
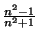
converge a
l = 1. En efecto, dado

> 0, veamos
qué necesitamos para que
 an
an - 1

<

:
Para que esto ocurra basta que
n2 >

, esto es
n >
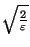
. Entonces basta con tomar
N =
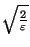
![$ \left.\vphantom{
\sqrt{\frac{2}{\varepsilon}}}\right]$](img193.gif)
+ 1.
Ejemplo 2.6.15
La sucesión
((- 1)
n) diverge. Para demostrar esto, supongamos lo
contrario, y denotemos su límite por
l. Tomando

= 1
tendríamos la existencia de
N tal que
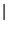
(- 1)
n -
l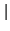
< 1,
para todo
n 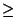 N
N. Pero para
n par obtenemos
l > 0, y para
n impar
obtenemos
l < 0, lo cual es contradictorio.
El siguiente lema nos permite hablar de ``el límite'' de una sucesión.
Lema 2.6.1
Si (an) converge, su límite es único.
Prueba
Supongamos que l1 y l2 son límites de (an). Sea
 > 0. Por definición existen N1 y N2 tales que
> 0. Por definición existen N1 y N2 tales que
| | an - l1| |
< 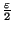 , ,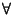 n n 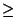 N1, N1, |
| | an - l2| |
< 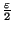 , ,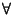 n n 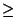 N2. N2. |
Tomando
n = max{N1, N2} se obtiene:
|
l1 -
l2| = |
l1 -
an +
an -
l2|

|
l1 -
an| + |
an -
l2| <
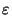
.
 para cada
para cada
 > 0,
con lo cual
l1 = l2.
> 0,
con lo cual
l1 = l2.
Otro resultado importante que resulta directo de la definición de lí
mite es el siguiente:
Lema 2.6.2
Toda sucesión convergente es acotada.
Prueba
Consideremos una sucesión convergente
 an
an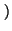 ,
y sea l su límite. Entonces tomando
,
y sea l su límite. Entonces tomando
 = 1, existe
N
= 1, existe
N 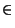 IN tal que
IN tal que
|
an| - |
l|

|
an -
l| < 1,
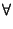 n
n 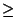 N
N.
M = max{| a1|,...,| aN - 1|,| l| + 1},
obtenemos
| an| 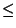 M para todo
n
M para todo
n 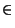 IN.
IN.
Nótese que el recíproco es falso, pues por ejemplo la
sucesión
((- 1)n) es acotada y no convergente.
La demostración del siguiente lema la dejaremos como ejercicio:
Lema 2.6.3
Supongamos que (an) y (bn) son sucesiones convergentes, tales que
para algún
N 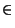 IN se tiene
IN se tiene
Entonces
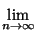 an
an 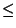
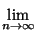 bn
bn.
En particular, si para cada n se tiene
an 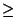 0, entonces
0, entonces
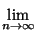 an
an 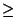 0.
0.
El siguiente resultado permite el cálculo y análisis de la
convergencia de ciertas sucesiones, con base en otras más simples. Este
resultado será central en la construcción de la función
exponencial que expondremos luego.
Teorema 3 (Ley del emparedado)
Supongamos que las sucesiones (bn) y (cn) son
convergentes a l, y que existe n0 tal que
bn 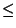 an
an 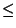 cn,
cn,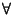 n
n 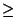 n0. Entonces (an) también converge a l.
n0. Entonces (an) también converge a l.
Prueba
Dado
 > 0, existe
n1
> 0, existe
n1 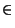 IN tal que
| bn - l| <
IN tal que
| bn - l| <  ,
,
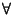 n
n 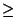 n1, y existe n2 tal que
n1, y existe n2 tal que
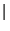 cn - l
cn - l <
<  ,
,
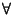 n
n 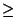 n2. Definiendo
N = max
n2. Definiendo
N = max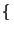 n0, n1, n2
n0, n1, n2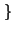 tenemos
tenemos
l -
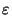
<
bn  an
an  cn
cn <
l +
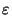
,
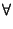 n
n 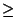 N
N.
Por lo tanto (an) converge a l.
Ejemplo 2.6.16
La sucesión
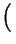

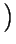 converge a 0, pues
-
converge a 0, pues
- 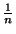
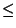

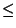
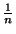 ,
,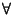 n
n 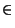 IN.
IN.
Ejemplo 2.6.17
Considere la sucesión
an = ![$ \sqrt[n]{2}$](img205.gif) . Tomando
. Tomando
 =
= ![$ \sqrt[n]{2}$](img205.gif) - 1 > 0, tenemos por la desigualdad de Bernoulli (ejercicio
3) que
- 1 > 0, tenemos por la desigualdad de Bernoulli (ejercicio
3) que
Esto muestra que
Por el teorema del emparedado se sigue que

0, y
consecuentemente
an =
![$ \sqrt[n]{2}$](img205.gif)
1.
Si
an  l
l  0, entonces eventualmente debe tenerse
an
0, entonces eventualmente debe tenerse
an 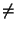 0. Mejor aún, an se mantiene lejos de cero, para n grande. El
siguiente lema expresa este hecho, de gran utilidad teórica.
0. Mejor aún, an se mantiene lejos de cero, para n grande. El
siguiente lema expresa este hecho, de gran utilidad teórica.
Lema 2.6.4
Supongamos que
an 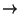 l
l 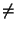 0. Entonces existe n0 tal que para
cada
n
0. Entonces existe n0 tal que para
cada
n 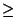 n0 se tiene
n0 se tiene
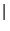 an
an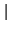 >
> 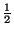
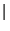 l
l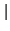 .
.
Prueba
Basta tomar
 =
= 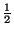
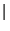 l
l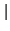 , el cual es positivo,
y aplicar la definición de convergencia. Obtenemos la existencia de
n0 tal que
, el cual es positivo,
y aplicar la definición de convergencia. Obtenemos la existencia de
n0 tal que
l -
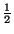
 l
l
<
an <
l +
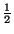
 l
l
,
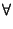 n
n 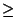 n0
n0.
Si l > 0 esto implica
an > l - 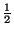 l =
l = 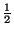 l. si por el
contrario l < 0, se obtiene
an < l +
l. si por el
contrario l < 0, se obtiene
an < l + 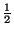
 - l
- l =
= 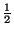 l.
l.
El cálculo de límites de sucesiones, se simplifica enormemente con el
uso del siguiente teorema:
Prueba
- 1.
- Dado
 > 0, aplicamos la definición de convergencia de
> 0, aplicamos la definición de convergencia de
 an
an , con
, con
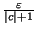 en
lugar de
en
lugar de
 , obteniendo que existe N tal que
, obteniendo que existe N tal que
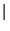 an - l
an - l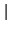 <
< 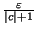 , para n
, para n 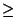 N.
Luego
N.
Luego
- 2.
- Dado
 > 0, existen n0 y n1 tales que
> 0, existen n0 y n1 tales que
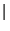 an
an -
a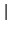
<
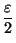
,
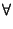 n
n 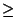 n0
n0;
 bn
bn -
b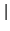
<
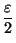
,
 n
n 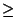 n1
n1.
Tomando
N = max n0, n1
n0, n1 obtenemos que
Esto demuestra que
an + bn
obtenemos que
Esto demuestra que
an + bn 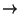 a + b. Además, por la parte
1 tenemos que
c . an
a + b. Además, por la parte
1 tenemos que
c . an 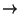 c . a, y por lo que
acabamos de demostrar se obtiene que
c . an + bn
c . a, y por lo que
acabamos de demostrar se obtiene que
c . an + bn 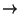 c . a + b.
c . a + b.
- 3.
- Como (bn) es convergente, se sigue que es acotada, y entonces
existe T > 0 tal que
| bn|
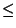 T,
T,
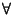 n
n 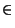 IN. Además
IN. Además
|
anbn -
ab| =
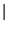
 an
an -
a bn
bn +
a bn
bn -
b

|
an -
a||
bn| + |
a||
bn -
b|,
|
anbn -
ab|
 T
T an
an -
a
+ |
a|
 bn
bn -
b
.
 an - a
an - a
 0 y
0 y
 bn - b
bn - b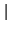
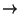 0, las propiedades anteriores permiten concluir que
Por el teorema del emparedado concluimos que
0, las propiedades anteriores permiten concluir que
Por el teorema del emparedado concluimos que
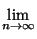
|
anbn -
ab| = 0.
- 3.
- Por el lema anterior, existe n0 tal que
| bn| >
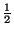 | b|,
| b|,
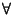 n
n 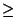 n0. Luego
Por la propiedad 1 y el teorema del emparedado concluimos que
Combinando con la propiedad 2, obtenemos
n0. Luego
Por la propiedad 1 y el teorema del emparedado concluimos que
Combinando con la propiedad 2, obtenemos
Ejemplo 2.6.18
Sea
an = 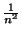 . Como
. Como
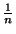
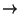 0, tenemos que
0, tenemos que
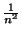 =
= 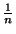 .
. 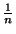
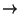 0, por la ley del producto.
0, por la ley del producto.
Ejemplo 2.6.19
Si
an = 1 +  -
-  tenemos que
an
tenemos que
an 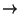 1, por
el ejemplo anterior, y las propiedades 1, 2 y 4.
1, por
el ejemplo anterior, y las propiedades 1, 2 y 4.
Ejemplo 2.6.20
Si
an = 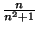 tenemos
an =
tenemos
an = 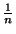 .
. 
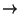 0 .
0 . 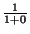 = 0.
= 0.
Ejemplo 2.6.21
Sea
an = 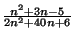 . Factorizando n2 tenemos
. Factorizando n2 tenemos
Ejemplo 2.6.22
Considere la sucesión
an = ![$ \sqrt[n]{a}$](img126.gif) , donde a > 1
es fijo. Tomando
, donde a > 1
es fijo. Tomando
 =
= ![$ \sqrt[n]{a}$](img126.gif) - 1 > 0, tenemos por la desigualdad
de Bernoulli (ejercicio 3) que
- 1 > 0, tenemos por la desigualdad
de Bernoulli (ejercicio 3) que
Esto demuestra que
Por la propiedad 1 del teorema anterior se tiene que
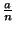
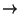
0, y por el teorema del emparedado se concluye que

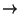
0. Consecuentemente, por la propiedad 2 del teorema anterior
se concluye:
Ejemplo 2.6.23
Si
an = 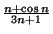 tenemos
tenemos
Además
Por el teorema del emparedado obtenemos que
an 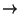
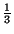
.
El siguiente teorema será la base en la construcción de la función
exponencial que haremos luego.
Teorema 5 (Weierstrass)
Toda sucesión monótona y acotada es convergente.
Prueba
Sea (an) acotada y creciente. Sea
l = sup{an : n 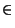 IN}, y sea
IN}, y sea
 > 0. Por definición de supremo (o el
teorema 1) existe
N
> 0. Por definición de supremo (o el
teorema 1) existe
N 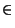 IN tal que
aN > l -
IN tal que
aN > l -  .
Como
.
Como
 an
an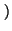 es creciente se sigue que
es creciente se sigue que
Esto demuestra que
an 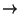 l. El caso decreciente se trata de
manera similar.
l. El caso decreciente se trata de
manera similar.
Ejemplo 2.6.24
Considere la sucesión dada recurrentemente por:
Los términos de esta sucesión son
Veremos que (
an) es creciente y acotada superiormente. Para esto
probaremos, usando el principio de inducción, que para todo
n 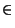 IN
IN se tiene
an 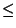 an + 1
an + 1 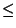
2.
Luego, por el teorema de Weierstrass tenemos que existe
l 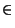 IR tal que
an
IR tal que
an 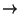 l. Pero por las propiedades de los
límites se debe tener
l. Pero por las propiedades de los
límites se debe tener
y entonces
l2 -
l - 2 = 0. Esto demuestra que
l = - 1 ó
l = 2, pero como
l es positivo, se concluye que
l = 2. Hemos demostrado que
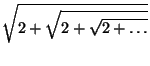
= 2.
Note que el teorema de Weierstrass es imprescindible en este
ejemplo, ya que la manipulación para hallar l solo está justificada
después de conocer su existencia.
![]() an
an![]() converge a l. Para precisar esto
mejor, tomamos un intervalo
]l -
converge a l. Para precisar esto
mejor, tomamos un intervalo
]l - ![]() , l +
, l + ![]() [, con
[, con
![]() > 0. Si los an se acercan a l, debe tenerse
an
> 0. Si los an se acercan a l, debe tenerse
an ![]() ]l -
]l - ![]() , l +
, l + ![]() [, para n suficientemente grande. Esto
justifica la siguiente definición.
[, para n suficientemente grande. Esto
justifica la siguiente definición.
![]() > 0 existe
N
> 0 existe
N ![]() IN tal que
IN tal que
![]() > 0. Debemos hallar N de manera que
> 0. Debemos hallar N de manera que
![]() <
< ![]() para todo n
para todo n ![]() N. Esto es equivalente a tener
n >
N. Esto es equivalente a tener
n > ![]() , para n
, para n ![]() N. Entonces basta tomar
N =
N. Entonces basta tomar
N = ![]()
![]()
![]() + 1, veamos:
+ 1, veamos:
 - 1
- 1![]() > 0. Por definición existen N1 y N2 tales que
> 0. Por definición existen N1 y N2 tales que
![]() ,
,![]() n
n ![]() N1,
N1,![]() ,
,![]() n
n ![]() N2.
N2.![]() an
an![]() ,
y sea l su límite. Entonces tomando
,
y sea l su límite. Entonces tomando
![]() = 1, existe
N
= 1, existe
N ![]() IN tal que
IN tal que
![]() 0, entonces
0, entonces
![]() an
an ![]() 0.
0.
![]() > 0, existe
n1
> 0, existe
n1 ![]() IN tal que
| bn - l| <
IN tal que
| bn - l| < ![]() ,
,
![]() n
n ![]() n1, y existe n2 tal que
n1, y existe n2 tal que
![]() cn - l
cn - l![]() <
< ![]() ,
,
![]() n
n ![]() n2. Definiendo
N = max
n2. Definiendo
N = max![]() n0, n1, n2
n0, n1, n2![]() tenemos
tenemos
![]() l
l ![]() 0, entonces eventualmente debe tenerse
an
0, entonces eventualmente debe tenerse
an ![]() 0. Mejor aún, an se mantiene lejos de cero, para n grande. El
siguiente lema expresa este hecho, de gran utilidad teórica.
0. Mejor aún, an se mantiene lejos de cero, para n grande. El
siguiente lema expresa este hecho, de gran utilidad teórica.
![]() =
= ![]()
![]() l
l![]() , el cual es positivo,
y aplicar la definición de convergencia. Obtenemos la existencia de
n0 tal que
, el cual es positivo,
y aplicar la definición de convergencia. Obtenemos la existencia de
n0 tal que
![]() l =
l = ![]() l. si por el
contrario l < 0, se obtiene
an < l +
l. si por el
contrario l < 0, se obtiene
an < l + ![]()
![]() - l
- l![]() =
= ![]() l.
l.
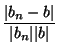 <
< 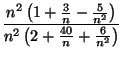 =
= 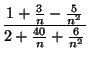
![]() IN}, y sea
IN}, y sea
![]() > 0. Por definición de supremo (o el
teorema 1) existe
N
> 0. Por definición de supremo (o el
teorema 1) existe
N ![]() IN tal que
aN > l -
IN tal que
aN > l - ![]() .
Como
.
Como
![]() an
an![]() es creciente se sigue que
es creciente se sigue que
 ,
, ,....
,....
![]() IR tal que
an
IR tal que
an ![]() l. Pero por las propiedades de los
límites se debe tener
l. Pero por las propiedades de los
límites se debe tener
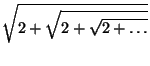 = 2.
= 2.